Perspective shape from shading and viscosity solutions
Résumé
This article proposes a solution of the Lambertian shape from shading (SFS) problem in the case of a pinhole camera model (performing a perspective projection). Our approach is based upon the notion of viscosity solutions of Hamilton-Jacobi equations. This approach allows us to naturally deal with nonsmooth solutions and provides a mathematical framework for proving correctness of our algorithms. Our work extends previous work in the area in three aspects. First, it models the camera as a pinhole whereas most authors assume an orthographic projection, thereby extending the applicability of shape from shading methods to more realistic images. Second, by adapting the brightness equation to the perspective problem, we obtain a new partial differential equation (PDE). Results about the existence and uniqueness of its solution are also obtained. Third, it allows us to come up with a new approximation scheme and a new algorithm for computing numerical approximations of the ?continuous? solution as well as a proof of their convergence toward that solution.
Fichier principal
 prados-faugeras-iccv03.pdf (760.35 Ko)
Télécharger le fichier
prados-faugeras-iccv03.pdf (760.35 Ko)
Télécharger le fichier
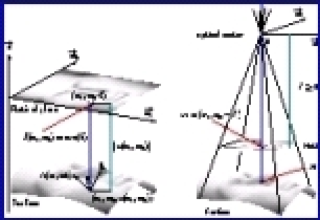 image-ICCV03.jpg (16.94 Ko)
Télécharger le fichier
image-ICCV03.jpg (16.94 Ko)
Télécharger le fichier
Origine : Fichiers produits par l'(les) auteur(s)
Format : Figure, Image
Loading...