Multiresolution morphing for planar curves
Résumé
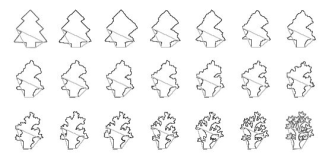
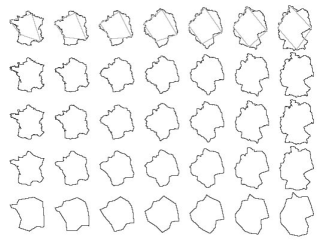
We present a multiresolution morphing algorithm using "as-rigid-as-possible" shape interpolation combined with an angle-length based multiresolution decomposition of simple 2D piecewise curves. This novel multiresolution representation is defined intrinsically and has the advantage that the details' orientation follows any deformation naturally. The multiresolution morphing algorithm consists of transforming separately the coarse and detail coefficients of the multiresolution decomposition. Thus all LoD (level of detail) applications like LoD display, compression, LoD editing etc.\ can be applied directly to all morphs without any extra computation. Furthermore, the algorithm can robustly morph between very large size polygons with many local details as illustrated in numerous figures. The intermediate morphs behave natural and least-distorting due to the particular intrinsic multiresolution representation.
Fichier principal
 HBCC07-computing.pdf (596.39 Ko)
Télécharger le fichier
HBCC07-computing.pdf (596.39 Ko)
Télécharger le fichier
 IMG1.jpg (29.22 Ko)
Télécharger le fichier
IMG1.jpg (29.22 Ko)
Télécharger le fichier
 IMG2.jpg (41.68 Ko)
Télécharger le fichier
IMG2.jpg (41.68 Ko)
Télécharger le fichier
Origine : Fichiers produits par l'(les) auteur(s)
Format : Figure, Image
Format : Figure, Image